7. Step 7: Estimate cross-model and network effects
Steps
7.1 Determine whether cross-modal and network effects matter.
7.2 Estimate benefits or disbenefits on related infrastructure due to diverted and generated traffic.
7.3 Consider application in complex urban networks
7.4 If the initiative results in changes to land use change, there may be additional benefits
7.5 If future investments in related infrastructure are deferred (brought forward), estimate net benefits (disbenefits)
7.1 Determine whether cross-modal and network effects matter
Refer to this section when undertaking a CBA of an initiative that alters the use of other transport infrastructure (in addition to use of the infrastructure created or improved by the initiative being appraised), regardless of mode.
Determine which of these two categories the effect fits into:
-
Diverted demand (substitution) – where passengers or freight switch from parallel infrastructure to the infrastructure created or improved by the initiative being appraised (e.g. a rail upgrade that attracts freight from road, a road improvement that reduces traffic on alternative routes)
- or -
-
Upstream/downstream effects (complementarity) – where additional use of infrastructure created or improved by the initiative being appraised also causes increased use of upstream or downstream infrastructure (such as a road or rail upgrade that results in additional usage in other parts of the route or at terminals).
See Figure 1 for an illustration of the concepts defined here.
Figure 1: Related infrastructure and traffic changes
7.2 Estimate benefits or disbenefits on related infrastructure due to diverted and generated traffic
This section discusses benefit estimation on related infrastructure, either parallel infrastructure or upstream/downstream infrastructure. Boxes 9 and 10 provide diagrammatic expositions, and boxes 11 and 12 numerical examples, that will assist readers in understanding the text below. It is suggested the text and the boxes be read concurrently.
Compare the perceived cost incurred by transport users with the marginal social generalised cost for the related infrastructure with altered demand. If they are practically the same, there are no further benefits or costs to consider. Note that where the related infrastructure is a congested road, the absence of congestion pricing may result in the perceived cost being below the social generalised cost.
Where traffic on the related infrastructure is lower in the Project Case than the Base Case, and the marginal social generalised cost is above (below) the marginal perceived cost, there will be an additional benefit (disbenefit). Normally, for parallel infrastructure, the traffic will be lower in the Project Case because the quantity of traffic diverted away from the parallel infrastructure in the Project Case will outweigh any positive impact of any generated traffic.
Where traffic on the related infrastructure is higher in the Project Case than the Base Case, and the marginal social generalised cost is above (below) the marginal perceived cost, there will be an additional disbenefit (benefit). This will normally be the case for upstream and downstream infrastructure.
If costs are constant with respect to traffic level (that is, there are no changes in costs as a result of reduced congestion), then:
additional benefit = (perceived [average] cost – average social generalised cost ) ×
change in quantity of traffic on the related infrastructure
Whether the result is positive (a benefit) or negative (a disbenefit) depends on the signs of the two terms in the formula. The difference between perceived and social costs is positive if the perceived cost exceeds social cost, as would be the case for a tax. The difference in costs would be negative for a subsidy or if transport users failed to perceive some of the costs they incur.
The change in the quantity of traffic would be positive for an increase and negative for a decrease. Hence, careful application of formula, ensuring the signs of the two terms are correct, will ensure the correct sign for the result.
If costs fall (increase) as a result of reduced (increased) congestion on the related infrastructure, the benefit is still the area between the average perceived cost curve and the social generalised cost curve over the quantity change. For social generalised costs, the marginal social cost curve must be used as it includes the congestion externality. For small changes, linear approximations of the cost curves can be used. For both the perceived (average) cost and marginal social generalised cost, obtain approximations by taking the halfway point between the Base Case and Project Case values[1].
When projecting social and private costs of related infrastructure into the future, adjust them upward for increasing congestion due to traffic growth over time and downward for cost reductions due to likely expansions of, or improvements to, the related infrastructure. Allow for feedback effects on the quantity of diverted traffic.
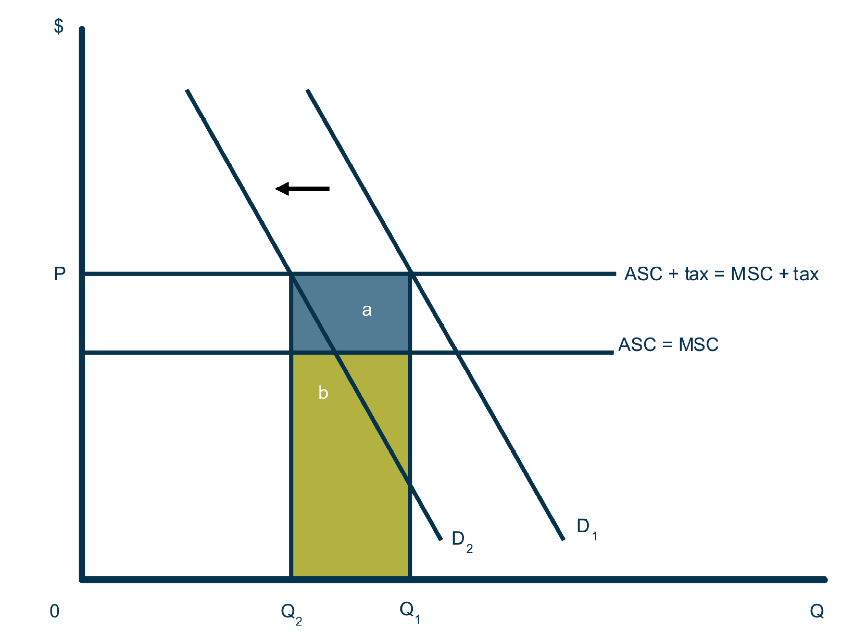
Box 9: Diagrammatic explanation of benefit estimation on parallel infrastructure: price > cost
The diagram shows the case where perceived cost exceeds social generalised cost on parallel infrastructure - for example, due to the fuel excise. Costs are assumed to be constant, so average social cost (ASC) equals marginal social cost (MSC), and there are no other distortions. The perceived price (P) is average cost plus the tax. The initiative induces a leftward shift in the demand curve from D1 to D2 causing the quantity of traffic to fall from Q1 to Q2. For each unit of demand, users give up P in WTP and society saves ASC = MSC. Because P > MSC, the loss of WTP exceeds the resource cost saving so there is negative benefit. The full loss of WTP is the sum of the rectangular areas a and b and the saving in resource costs is area b. The net disbenefit is therefore area a. The disbenefit is borne by the government in the form of lost tax revenue.
The negative result is consistent with the formula in Section 7.2 because the quantity change is negative. Had the demand curve shifted right, as may occur for upstream or downstream infrastructure, the subscripts, 1 and 2, for the demand curves and quantities would be reversed and area a would be a benefit. The government would gain tax revenue equal to area a.
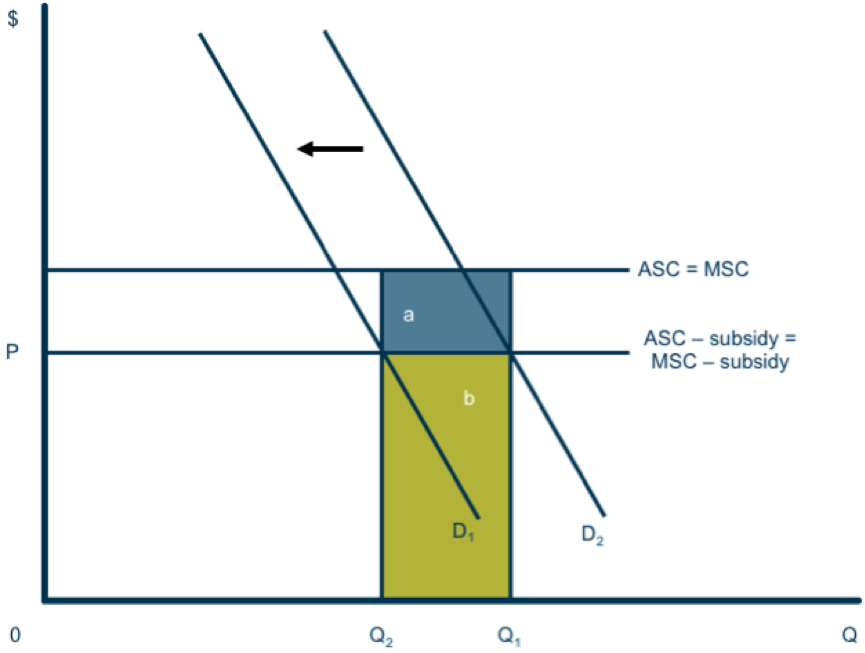
Box 10: Diagrammatic explanation of benefit estimation on parallel infrastructure: cost > price
The diagram shows the case where social generalised cost exceeds perceived cost on parallel infrastructure, for example, due to a subsidy as is often the case for public transport. Costs are assumed to be constant, so average social cost (ASC) equals marginal social cost (MSC), and there are no other distortions. The perceived price (P) is average social minus the subsidy. The initiative induces a leftward shift in the demand curve from D1 to D2 causing the quantity of traffic to fall from Q1 to Q2. For each unit of demand, users give up P in WTP and society saves ASC = MSC. Because MSC > P, the resource cost saving exceeds the loss of WTP so there is a net benefit. The full resource saving is loss of WTP is the sum areas a and b. The net benefit is area a. The benefit accrues to the government in the form of a saving in the amount of subsidy it has to pay.
In terms of the formula in Section 7.2, both terms are negative, which cancel out to give positive result.
Another reason why social costs could exceed perceived costs is failure of users to perceive part of the costs they incur. In this case, the benefit, area a, would accrue to users. For example, if they made travel decisions treating car running costs as a fixed charge per period of time, in the project case, they would find themselves paying less run their vehicles.
Had the demand curve shifted right, as would occur for upstream or downstream infrastructure, the subscripts for the demand curves and quantities would be reversed and area a would be a disbenefit. The government would have pay an increased amount of subsidy, or car users failing to perceive car running costs for find themselves having to pay more to run their vehicles.
Boxes 11 and 12 illustrate the above discussion with examples of the estimation of benefits from diverted traffic, and upstream and downstream traffic, respectively.
Box 11: Numerical examples of estimation of benefits from diverted traffic
A rail infrastructure upgrading initiative results in a diversion of 1000 tonnes of freight per annum from road transport to rail.
Without congestion
The perceived cost by road for the door-to-door task is $90 per tonne. The social generalised cost of the door-to-door movement by road over the route is $100 per tonne. There is an annual benefit of ($90 – $100) x –1000 tonnes = $10 000.
If the perceived cost by road is $105 per tonne – above the social generalised cost – the annual benefit is negative: ($105 – $100) x –1000 tonnes = –$5000, a disbenefit of $5000.
With congestion (linear approximation of cost curves)
Say the road is congested. The Base Case perceived cost and marginal social generalised cost are $90 and $100 respectively. Due to reduced congestion on the road following the loss of traffic, the Project Case perceived cost and marginal social generalised cost are $86 and $94 respectively. The average (halfway point) of the Base Case and Project Case perceived costs is ($86 + $90)/2 = $88. The average (halfway point) of the Base Case and Project Case marginal social generalised costs is ($100 + $94)/2 = $97. The benefit from traffic diversion is then ($88 – $97) x –1000 tonnes = $9000.
Note that the same benefit can be calculated as the reduction in average social generalised cost to users who remain on the road plus a benefit for diverting users obtained by applying the rule-of-half. See NGTSM 2006, Volume 5, Section 2.7, Example K for an explanation.
Box 12: Numerical examples of estimation of costs from upstream-downstream effects
A rail infrastructure upgrading initiative results in a diversion of 1000 tonnes of freight per annum from road transport to rail.
Without congestion
The perceived cost of carrying the freight on the feeder road is $9 per tonne. The social generalised cost is $10 per tonne. Using the formula in Section 7.2, there is an annual benefit of ($9 – $10) x 1000 tonnes = $1000, a disbenefit of $1000.
If the perceived cost by road is $11 per tonne – above the social generalised cost – there is an annual benefit: ($11 – $10) x 1000 tonnes = $1000.
With congestion (linear approximation of cost curves)
Say the road is congested. The Base Case perceived cost and marginal social generalised cost are $8 and $10 respectively. Due to increased congestion on the road following the increase in traffic, the Project Case perceived cost and marginal social generalised cost are $12 and $14 respectively. The average of the Base Case and Project Case perceived cost is ($8 + $12)/2 = $10. The average of the Base Case and Project Case marginal social generalised cost is ($10 + $14)/2 = $12. The benefit from traffic diversion is then ($10 – $12) x 1000 tonnes = $2000, a disbenefit of $2000.
7.3 Application in complex urban networks
7.3.1 Measuring user benefits
The discussion in Sections 7.1 to 7.2 presented the principles for estimating user benefits when cross-modal and network effects apply. Direct application of the above discussion is feasible in cases where those cross-modal and network effects are relatively simple. When the network and the associated cross-effects are complex, the assessment also becomes much more complex. This is the case for the urban networks of cities.
The complexity of travel patterns is illustrated as follows:
- There are multiple routes throughout the city
- Those routes can be parallel and also cross each other
- There are multiple transport modes that can be chosen: car, car pooling, public transport (bus, train, tram), cycling, walking
- Activities are scattered across the urban area and also concentrated in centres
- Patterns of localised traffic and through traffic is repeated across many sub-areas within the city.
All these features of cities produce a vast range of travel options and choices for users between many origins and many destinations, dispersed across a metropolitan area. In these complex urban cases, the estimation of travel decisions and user benefits requires the use of more sophisticated analytical methods, namely those available through urban travel demand models[2]. Part T1 of the ATAP Guidelines provides guidance on travel demand models.
The measurement of user benefits using travel demand models still involves use of the same principles discussed in the above sections. Equations (1), (3) and (4) in Sections 6.2 and 6.3 define the user benefit for existing traffic, new traffic and both traffics combined. Section 7.4 produced the same user benefit equations using the alternative method of consumer surplus plus resource correction.
However, the difference with complex urban networks is in how the formulas are applied:
- The user benefit calculations first need to be undertaken within the travel demand model at a disaggregated level: for each origin-destination pair[3], for each mode, for each time period and for each forecast year
- The disaggregated results are then aggregated to yield overall use benefits:
- Aggregating across the entire demand matrix (that is, across all origin-destination pairs)
- Repeating the process for all modes and time periods
- Repeating the process for each model forecast year.
With a highly disaggregated base, a wide range of user benefit breakdowns can be summarised to facilitate a good understanding by both the analysts and the decision-maker of how user benefits are expected to vary by time periods, by mode, by geographical location and by forecast year.
7.3.2 Accounting for induced demand
Part T1 Section 3.4 of the ATAP Guidelines discusses induced demand[4]. It states that induced demand refers to the impacts of transport improvements in encouraging some people to switch routes, modes or time of travel to take advantage of the improved travel times and service levels. In addition, induced demand can refer to the tendency of some people to travel more, or travel further, when travel conditions are improved. In the demand model, induced demand can arise from changes in any of the following: route choice, time of day travel occurs, mode choice, trip distribution (that is, choice of trip destination), trip generation (that is, the number of trips undertaken), land use changes and the location decisions of both households and businesses.
In economic terms, the induced (additional) traffic resulting from, say, a road network improvement will perceive a benefit through now being able to travel, taking advantage of the improved conditions. However, this additional traffic will reduce the potential benefits of the improvements for other traffic if the road network is at all congested.
The inclusion of induced demand effects can make a significant difference to user benefit estimates. For example, research a couple of decades ago (Huw et al, 1992) found that failure to account for induced demand overvalued road capacity expansion benefits by 50 per cent or more. Other studies (Abelson and Hensher, 2001 and Litman, 2008) have also found that excluding induced demand can materially overstate the economic benefits of an initiative.
Given the significant potential impact of induced demand, best practice in the assessment of major urban transport initiatives now requires that the outputs from the demand model (both travel estimates and user benefit estimates) take account of induced demand.
For example, in the case of major urban road initiatives, it is not sufficient to assume that the only difference between Base Case and Project Case numbers of peak period users will arise from users switching routes to take advantage of improved speeds on the initiative route. Such an approach ignores the complexity of real-world responses to major transport investments (Bray, 2005).
Induced demand is only expected to be of material significance for large urban transport initiatives. Induced travel demand effects are of greatest importance for the assessment of transport initiatives in networks with:
- A high degree of congestion (typically in urban areas, especially at peak periods) and/or
- High elasticity of demand (typically in urban areas, especially where alternative modes offer strong competition) and/or
- Relatively large changes in travel costs (typically for larger schemes providing substantially enhanced capacity).
For public transport network improvements, induced demand effects are also most significant when similar conditions apply that is, when demand is elastic and increases in response to improved service, and when the service is already congested or crowded.
For major urban transport initiatives where induced demand is considered to be relevant to the assessment, Part T1 (Section 3.4.3) indicates that the Variable Trip Matrix (VTM) approach must be used in the demand modelling and associated user benefit calculations that accommodate the various sources of induced demand.
Finally, the case of large city-shaping transport initiatives should be specifically mentioned. Part F0.2 of the ATAP Guidelines discusses such initiatives, noting their significant potential impacts on land use and urban structure. Induced demand in such cases is therefore of high importance.
Part F0.2 explores the ideal of using a ‘fully evolved CBA’ of large city-shaping urban transport projects, with full modelling of land use-transport interaction. It notes, however, that there continue to be challenges to implementing such an approach at this point in time[5]. It suggests that a practical alternative approach is the iterative application of CBAs using land-use impact scenario analysis. In this approach, scenario analysis is used to investigate the potential major land-use impacts of strategic transport initiatives. Testing the effect of different land-use impact outcomes on a CBA determines the sensitivity of the CBA results.
7.4 If the initiative results in changes to land use change, there may be additional benefits
If the initiative results in more compact land use so there is less urban sprawl in the base, there may be some additional benefits to consider.
The same principal applies as outlined above in Sections 7.1 and 7.2. If prices (and hence changes in willingness-to-pay) equal marginal social costs, there are no additional net benefits. For example, if the households and businesses that locate on the urban fringes in the base case pay for the full resource cost of the additional land, infrastructure and services they require and the externalities they create, the resource cost is fully offset by the benefits to the land users.
There are only benefits to the extent that prices are below marginal social costs. For example, if the governments meet some of the costs of establishing and maintaining new outer suburbs in the base case, there is a net benefit from not having to create these suburbs in the project case. However, the benefit is limited to the difference between the resource cost and the private cost incurred by people who move to the new outer suburbs in the base case, not the full avoided resource cost of the creating the new suburbs. In other words, the benefit of the saving in the resource costs of creating and maintaining the new outer suburbs has to be reduced by the lost willingness-to-pay of the people who would have lived in those suburbs.
Not creating new outer suburbs in the Project Case may lead to some savings in congestion costs compared with the Base Case. These would be estimated in the usual way by assuming leftward shifts in the demand curves for the infrastructure affected in the Project Case. Lower externalities associated with transport to and from the fringe suburbs could be counted as benefits because they are unpriced. Loss of fuel excise to the government would count as a disbenefit, as the reduction in consumers' willingness-to-pay exceeds the resource cost saving.
Part T1 of the ATAP Guidelines provides guidance on travel demand modelling, including modelling of the interaction between land use and transport.
7.5 If future investments in related infrastructure are deferred (brought forward), estimate net benefits
The preceding sections asked analysts projecting social and private costs of related congested infrastructure into the future, to adjust their projections for cost reductions due to likely expansions of, or improvements to, the related infrastructure. A reduction in demand for use of related infrastructure can cause future expansions or improvements to be deferred, and, conversely, an increase in demand can cause future expansions to be brought forward in time. In discounted present value terms, deferral of future capital expenditure is a benefit and bringing of future capital expenditure is a cost.
If expansion and contraction of infrastructure capacity of related infrastructure was perfectly divisible and occurred in a way such that capacity was always optimal (capacity was adjusted so the marginal benefit of expansion was maintained equal to the marginal cost), there would no net benefits to consider from changes in the timing of future capacity changes on related infrastructure due to the initiative being appraised. The gains or losses from altered timings of future capital expenditures would be exactly offset by gains and losses to users of the infrastructure. Lumpiness in capacity expansion and over- or under-investment mean that changes in the timing of future capital expenditures on related infrastructure can give rise to additional impacts for inclusion in a CBA.
When estimating deferred infrastructure benefits or brought-forward infrastructure disbenefits, it is essential to offset them with any changes to user benefits on the related infrastructure. In some cases, the two offsetting impacts may approximately cancel out.
- If a reduction in demand on related infrastructure leads to a benefit from deferred capacity expansion, there will be an offsetting loss of benefit, during the deferral period, for users who remain on the related infrastructure.
- If an increase in demand on related infrastructure leads to a disbenefit from capacity expansion being brought forward in time, there will be an offsetting benefit, during the period over which the additional capacity has been brought forward, for existing users of the related infrastructure.
Changes in user benefits are estimated in accordance with the other sections in Chapters 6 and 7.
To note only – it does not affect the methodology: the impact on existing users of related infrastructure of a changed timing of capacity expansion will be greater if the planned capacity expansion is later than its optimal time, which would be indicated by a high benefit-cost ratio. Conversely, the impact on existing users of related infrastructure will be lower if the planned capacity expansion is before its optimal time, which would be indicated by a low benefit-cost ratio. In the extreme case, where the planned capacity expansion is not needed at all and creates zero user benefits, there will be no impact on existing users from changing its timing. There is only the change in the discounted cost of deferring or bringing forward the investment.
[1] An alternative measure of the benefit that is often used in practice is: Benefit = (APC1 - APC2) (Q2 + Q1)/2 +(APC – ASC)(Q2 - Q1) where APC1 and APC2 are average perceived costs in the Base and Project Cases respectively. The second term is a resource correction where APC = (APC1 + APC2)/2 and ASC = (ASC1 + ASC2)⁄2 where ASC is average social cost. Appendix A provides a technical proof.
[2] Travel demand models divide an urban area into a large number of smaller zones. Each zone is modelled as both an origin and a destination. Trips are modelled for every pair of origins and destinations across the urban area.
[3] In some practice, the calculations are ‘link-based’; that is they are undertaken for each link in the modelled transport network. The origin-destination approach discussed here is generally considered the best practice approach.
[4] Note that induced demand is equivalent to generated and diverted traffic, as discussed in earlier sections of Part T2.
[5] Section 3.5 of Part T1 provides an overview of the current state of land use-transport interaction modeling.